A spring having a spring constant of \(1200\) N/m is mounted on a horizontal table as shown in the figure. A mass of \(3\) kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of \(2.0\) cm and released. The frequency of oscillations will be:

1.
\(3.0~\text{s}^{-1}\)
2.
\(2.7~\text{s}^{-1}\)
3.
\(1.2~\text{s}^{-1}\)
4.
\(3.2~\text{s}^{-1}\)

The (displacement-time) graph of a particle executing SHM is shown in the figure. Then:
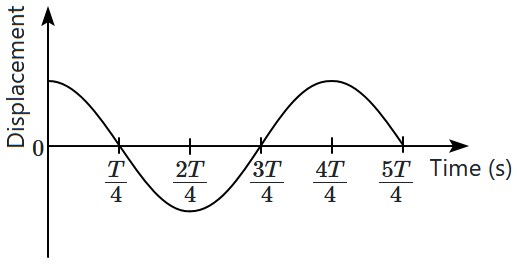
| (a) | the force is zero at \(t=\dfrac{3T}{4}\) |
| (b) | the acceleration is maximum at \(t=\dfrac{4T}{4}\) |
| (c) | the velocity is maximum at \(t=\dfrac{T}{4}\) |
| (d) | the potential energy is equal to the kinetic energy of oscillation at \(t=\dfrac{T}{2}\) |
| 1. | (a), (b) and (d) only | 2. | (a), (b) and (c) only |
| 3. | (b), (c) and (d) only | 4. | (c) and (d) only |
All the surfaces are smooth and the system, given below, is oscillating with an amplitude \({A}.\) What is the extension of spring having spring constant \({k_1},\) when the block is at the extreme position?

| 1. | \(\dfrac{k_1}{ k_1+k_2} A\) | 2. | \(\dfrac{k_2A}{k_1+k_2}\) |
| 3. | \(A\) | 4. | \(\dfrac{A}{2}\) |
The equation of a simple harmonic wave is given by \(y=3\sin \frac{\pi}{2}(50t-x)\) where \(x \) and \(y\) are in meters and \(t\) is in seconds. The ratio of maximum particle velocity to the wave velocity is:
| 1. | \(\frac{3\pi}{2}\) | 2. | \(3\pi\) |
| 3. | \(\frac{2\pi}{3}\) | 4. | \(2\pi\) |
The acceleration-time graph of a particle undergoing SHM is shown in the figure. Then,
| 1. | the velocity of the particle at point 2 is zero |
| 2. | velocity at point 3 is zero |
| 3. | velocity at point 2 is +ve and maximum |
| 4. | both (2) & (3) |
A particle is performing SHM with amplitude \(A\) and angular velocity \(\omega.\) The ratio of the magnitude of maximum velocity to maximum acceleration is:
1. \(\omega\)
2. \(\dfrac{1}{\omega }\)
3. \(\omega^{2} \)
4. \(A\omega\)
| 1. | a straight line and is periodic. |
| 2. | a circle and is non-periodic. |
| 3. | an ellipse and is periodic. |
| 4. | a parabola and is non-periodic. |
Simple harmonic motion is an example of:
| 1. | uniformly accelerated motion |
| 2. | uniform motion |
| 3. | non-uniform accelerated motion |
| 4. | all of the above |
The total mechanical energy of a linear harmonic oscillator is \(600~\text J.\) At the mean position, its potential energy is \(100~\text J.\) The minimum potential energy of the oscillator is:
1. \(50~\text J\)
2. \(500~\text J\)
3. \(0\)
4. \(100~\text J\)
When a periodic force \(\vec{F_1}\) acts on a particle, the particle oscillates according to the equation \(x=A\sin\omega t\). Under the effect of another periodic force \(\vec{F_2}\), the particle oscillates according to the equation \(y=B\sin(\omega t+\frac{\pi}{2})\). The amplitude of oscillation when the force (\(\vec{F_1}+\vec{F_2}\)) acts are:
| 1. | \(A+B\) | 2. | \(\sqrt{A^2+B^2}\) |
| 3. | \(\large\frac{\sqrt{A^2+B^2}}{2}\) | 4. | \(\sqrt{AB}\) |







